Published by: BhumiRaj Timalsina
Published date: 23 Jun 2021
The Schrodinger theory tells us how to obtain the wave function Ψ (x, t) associated with a particle, when we specify the forces acting on the particle, by giving the potential energy associated with the forces. (In quantum mechanics the potential energy is often referred to simply as the potential). The Schrodinger theory also tells us how to extract information about the particle from the associated wave function.
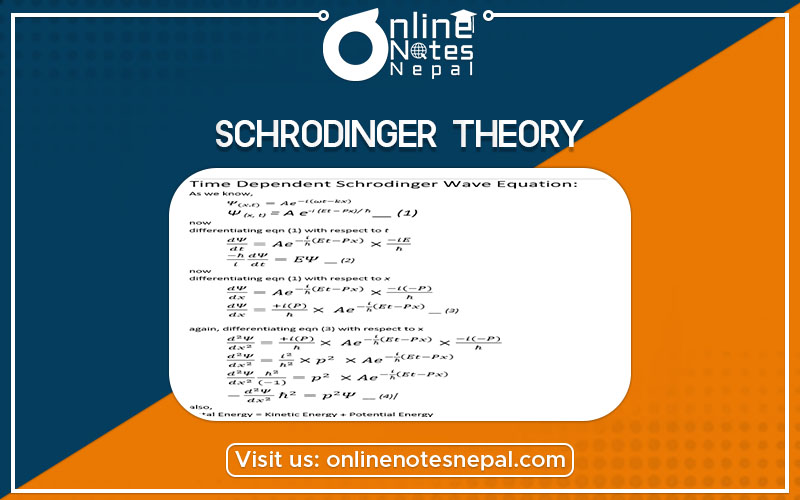
Schrodinger developed a differential equation whose solutions yield the possible wave functions that can be associated with a particle in a given physical situation. This equation, known as the Schrodinger equation, tells us how the wave function changes as a result of the forces acting on the particle.
Because the wave function Ψ is a function of space and time, the equation contains derivatives (remember that a derivative represents the rate of change) with respect to x, y, and z and with respect to t. In this chapter, we will primarily consider motion in only the x-direction. In mathematics, when a function depends on more than one independent variable, the derivative of the function with respect to one of them while treating the other variables as constants, is called the partial derivative with respect to that variable.
The partial derivative of Ψ with respect to x is written as dΨ/dx, with similar notation for the partial derivative with respect to t. Several plausibility arguments can be used to arrive at the Schrodinger equation. We present here one that is based on the idea, to be demonstrated later, that there is a relation between mathematical operators and physical quantities.
(In mathematics an operator is represented by a symbol or group of symbols and indicates an operation to be performed. Thus, for example, when the operator – i ћ d/dx is placed in front of a function, it indicates that the function is to be differentiated with respect to x and then multiplied by – i ћ, where i is the imaginary number √-1)
Equation
Equation
If you liked our content Schrodinger theory then please don’t forget to check our other topics Introduction