Schrodinger Equation for The H Atom

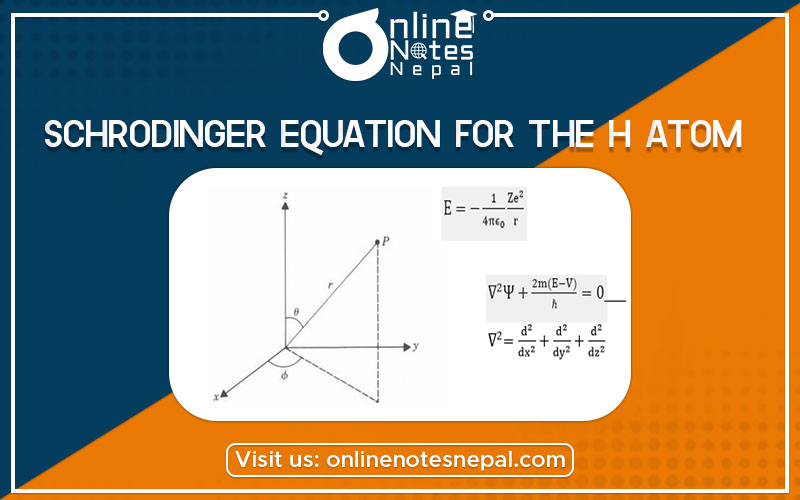
Schrodinger Equation for The H Atom deals about the Schrodinger theory on H atom. We saw in connection with the Bohr model of the hydrogen atom, that the potential energy of the electron in the electric field of a nucleus is

Equation

where r = (√(X2++ y2 + Z2) is the distance between the electron and the proton. If the nucleus has more than a single positive charge, we must include the additional charges in the Coulomb energy. We do this by a multiplicative term Z, called the atomic number, that represents the number of protons in the nucleus and also the number of electrons in neutral atoms. Thus the charge part of the Coulomb equation for a single electron becomes eZe or Ze2, and the above energy equation, which is for Z = I, is written as

Equation

We extend the time-independent Schrodinger equation to a situation where a particle is free to move in three dimensions. To do this we simply include derivatives of the space part of the wave function, X with respect to the other two coordinates, y, and z, namely,

Equation

the spherical polar coordinates are r, θ, and Φ

Photo

the rectangular coordinates are related to spherical polar coordinates:

x= r sinθ cos Φ

y= r sinθ sinΦ

z= r cosθ

therefore, the Schrodinger equation in the spherical polar coordinates is given by:

Equation

In this equation the wave function is the function of r, θ, and Φ.

let Ψ(r, θ, Φ) = R(r) G(θ) B(φ) __(4)

where

R(r) is the function of r only

G(θ) is the function of θ only

B(Φ.) is the function of Φ. only

now substituting this Eqn (4) in Eqn (3)

Equation

The left side of the above equation is a function of r and θ alone, whereas the right side is a function of φ alone. The only way the equation can be valid is when both sides of the equation are equal to the same constant. For convenience, we let this constant be – mL2, where this mL is not to be confused with the m used for mass. The right side of the equation gives us an ordinary differential equation for Φ.

Equation

Again, one side of this equation is a function of the variable r only, whereas the other side is a function of the variable θ only. The only way equality can be valid is when both sides are equal to the same constant. If we call this constant 1 (1 + 1), 1 will be an integer. We now get two differential equations, one for R and another for G.

Equation

the Schrodinger wave equation for the H atom have now separated into three equation each dependent on only one of the coordinates.

If you liked our content Schrodinger Equation for The H Atom then please don’t forget to check our other topics Application of The Schrodinger Theory