de-Broglie Hypothesis

de-Broglie Hypothesis says that all matter has both particle and wave nature. The wave nature of a particle is quantified by the de-Broglie wavelength. This was called a hypothesis because there was no evidence for it when it was proposed, only analogies with existing theories. (The wavelength-momentum relation holds exactly for photons).

The successful explanation of the Compton Effect, Photoelectric effect, and Blackbody radiation shows that electromagnetic radiation travels not only in the form of a continuous stream of energy but in the form of tiny packets or bundles of energy. These packets of energy were called “quanta”. For electromagnetic radiation these were called “photon”.

On the other hand, phenomena like Interference, Diffraction, and Polarization can’t be explained unless wave nature of electromagnetic radiation is assumed.

It was discovered that particles of atomic dimensions some times behave more like waves than discrete particles. It was also observed that electromagnetic waves sometimes exhibit properties similar to properties of discrete particles of matter.

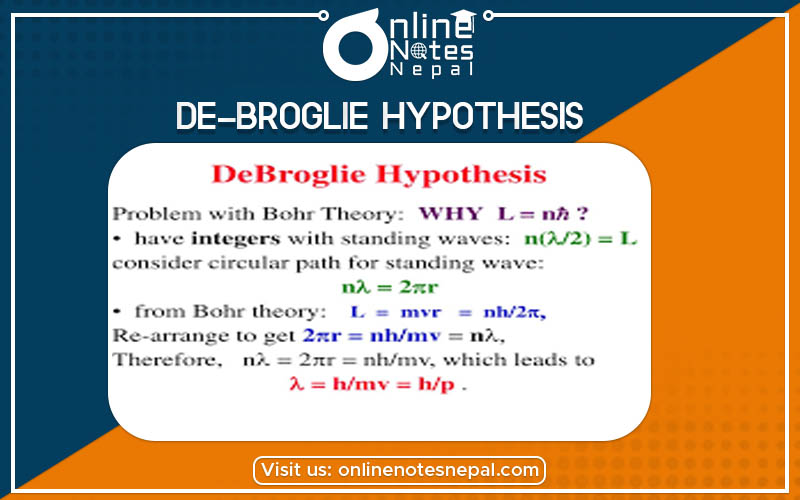
Louis de-Broglie in 1924 suggested his hypothesis that there is wave-particle dualism. The wave associated material particles is called “matter waves” or “pilot waves”.

To show the wave-particle dualism he mad the use of Planck’s theory of quantum radiation and Einstein’s theory of relativity.

According to the Planck’s theory of quantum radiation, the energy of the photon is given by;

E = hf = hc/λ     ……(1)

Again, from Einstein’s mass-energy relationship, the energy of the photon is given by;

E = mc2     ……….(2)

Comparing (1) and (2)

hc/λ = mc2

λ = h/mc = h/p

[where p = mc = momentum of photon]

According to the de-Broglie Hypothesis, the wavelength of the wave associated with the moving particle having momentum p = mv, is given by;

λ = h/mv   …….(3)

Experimental Verification of de-Broglie Hypothesis

To prove the de-Broglie hypothesis we have to show experimentally that a beam of particles exhibits wave-like properties such as interference and diffraction.

Consider a bullet of mass m = 0.1kg moving with velocity v = 10^3m/s. The wavelength of the wave associated with the bullet is;

λ = h/mv = 6.62×10-36 m

which is a very small wavelength. For diffraction and interference, the wavelength must be comparable to the size of slit or obstacle. It is next to impossible to obtain the slit or obstacle of size comparable to the above value. The most practical way to obtain the larger wavelength is to choose a particle of small mass as the electron.

Davisson and Germer observed that when a beam of electron strikes a single crystal of nickel, the intensity pattern is similar to that in the diffraction of light waves. The setup for Davisson and Germer experiment is as shown in figure(1).

Picture

Fig(1): Apparatus used in Davisson and Germer Experiment

Electrons from a heated filament are accelerated with a variable voltage V. The beam is made to incident on a single crystal of nickel. The intensity of the scattered beam can be measured by the detector for different angles and various voltages. While rotating, the detector observes the variation in intensity and the maximum intensity at a certain value as shown in the figure.

If the electrons only behave like particles for any incoming angle, we will observe the same number of particles rebounding at a similar angle regardless of their velocity and potential V. This will result in uniform intensity for different values of the angle. This proves the diffraction of the electron beam as a wave and is the direct experimental evidence of the de-Broglie Hypothesis.

If an electron of mass “m” is accelerated through the potential V and velocity ‘v’ then

½ mv2 = eV

V = 2eV/m

Therefore, the wavelength of the wave associated with the electron is;

λ = h/mv = h/(2meV)1/2

For the applied potential of V = 54V

λ = 6.62×10-36/(2×9.1×10-31×1.6×10-19×54)1/2

λ = 1.6×10-10 

Instead of electron, if we incident a beam of X-ray, the Bragg’s diffraction condition is;

2d sinθ = n λ

For Nickel, the atomic spacing is, d=0.91A

Here,

θ + ɸ + θ = 180 ֯

θ = 65 ֯

Picture

Now, for first-order(n=1),

λ = 2d sinθ/n

λ = 1.6×10-10 m

which is nearly equal to the wavelength of the electron beam. This agreement between the wavelength of the electron beam and X-ray shows that an electron beam produces the same diffraction pattern as produced by X-rays. Thus, the experiment confirms the de Broglie’s hypothesis that particles have wave properties.