Intrinsic Semiconductors

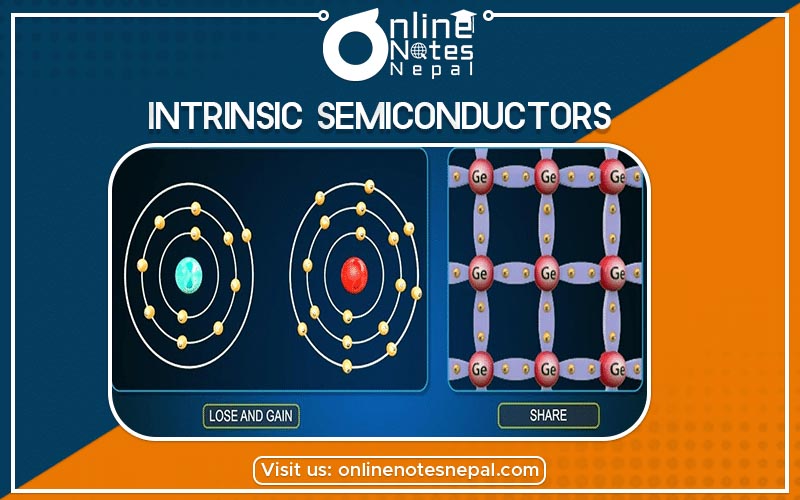
Intrinsic Semiconductors are those semiconductors whose impurity concentrations are so low that its electrical conductivity properties are not affected. They are solely determined by the band structure of the material itself. In an intrinsic semiconductor, at absolute zero temperature, it has a filled valence band and an empty conduction band. Hence, it acts as an insulator. There is no conduction of current. In-room temperature, there are enough electrons that get excited across the forbidden gap. This makes both electron and hole concentrations in the conduction and valence bands. The newly entered electron to the conduction band can move freely here and there in the solid namely free electrons. The space left behind is a hole.

Electron density in Intrinsic Semiconductor

In this type of semiconductor, there is a transfer of an electron from the valence band to the conduction band. It leaves the hole behind in the valence band. Then, the number of electrons in the conduction band is equal to the total number of holes in the valence band.

If ‘n’ is the density of electrons, i.e. the number of electrons per unit volume in the conduction band, then in the equilibrium condition,

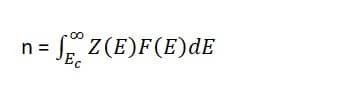
Where,

 

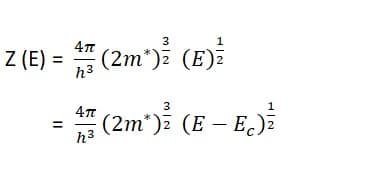
It is the density of the states in the conduction band. As the bottom of the conduction band has an energy Ec and not zero, we can substitute (E-Ec ) for E.

Now, the Fermi-Dirac distribution function is given by,

Now,

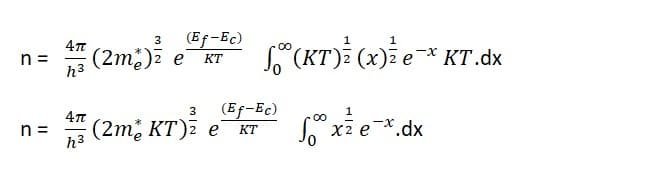
Using the integration table,

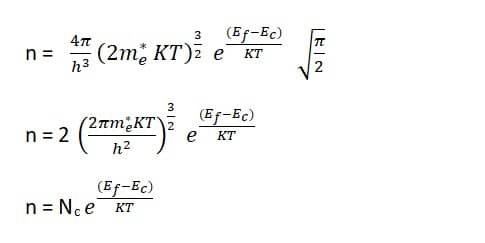
Where,

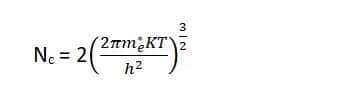
is the effective density of state at the conduction band edge.

 

Hole Concentration in Intrinsic Semiconductors

It is the state of energy in the valence band unoccupied by an electron. F (E) gives the probability of occupation for the energy states. There is the probability that it may be unoccupied by an electron is [1-F (E)]. It is the same as that it may be occupied by a hole.

Therefore, the concentration of holes in the valence band is,

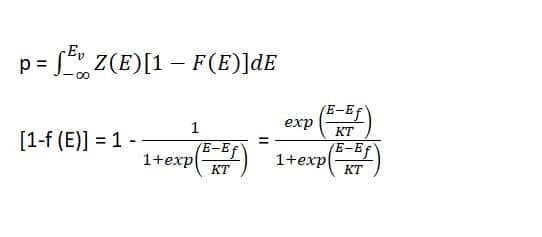
Here,

As E <

Therefore,

From the above equation, we see the value of [1-F (E)] i.e. the probability to find a hole decreases exponentially as we move from top to bottom of the valence band. This shows that holes reside near the top of the valence band.

Now, the value of Z (E) is,

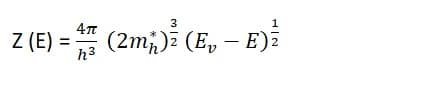
where, mh * is the effective mass of the hole.

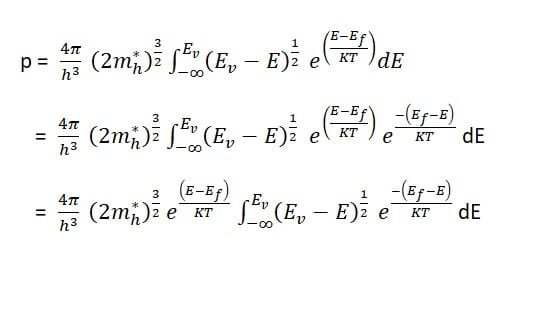
It is the effective density of the states at the valence band edge.

 

Fermi-Level in Intrinsic Semiconductor

For an intrinsic Semiconductor, the number of electrons in the conduction band ‘n’ is equal to the number of holes in the valence band p. This is because the electrons and holes are in pairs.

i.e.  n = p

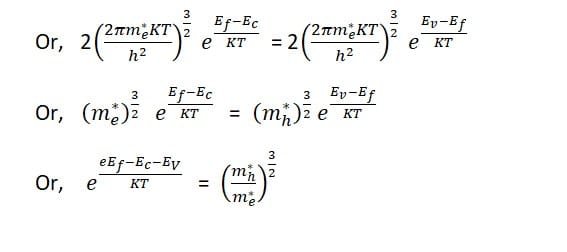
If we take natural log on both sides, we get,

At absolute zero temperature, the Fermi level lies exactly halfway between the top of the valence band and bottom of the conduction band.