Published by: Nuru
Published date: 25 Jun 2021
The free energy, which turns out to be the single most useful criterion for predicting the direction of a chemical reaction and the composition of the system at equilibrium. As we will explain near the bottom of this page, the term "free energy", although still widely used, is rather misleading, so we will often refer to it as "Gibbs energy."
In chemical thermodynamics, we prefer to focus our attention on the system rather than the surroundings and would like to avoid having to calculate the entropy change of the surroundings explicitly. The free energy enables us to do this for changes that occur at a constant temperature and pressure (the Gibbs energy) or constant temperature and volume (the Helmholtz energy.)
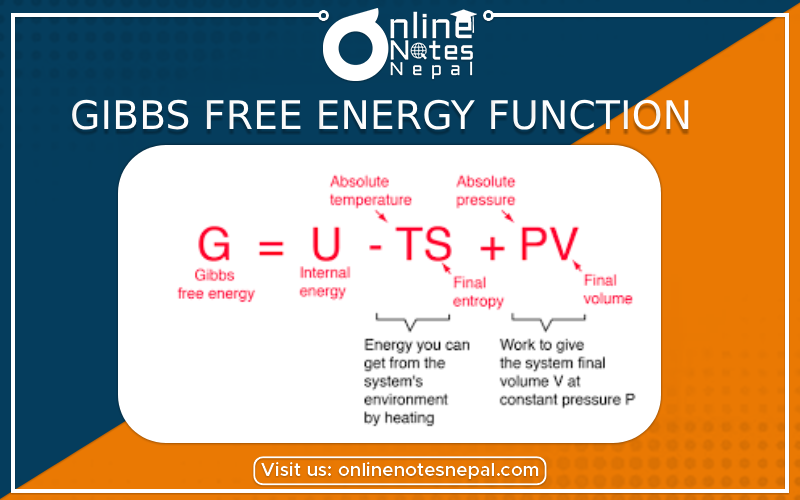
The Gibbs energy (also known as the Gibbs function) is defined as
G=H–TS
in which S refers to the entropy of the system. Since H, T and S are all state functions, so is G. Thus for any change in state (under constant temperature), we can write the extremely important relation
ΔG=ΔH–TΔS
How does this simple equation encompass the entropy change of the world ΔStotal, which we already know is the sole criterion for spontaneous change from the second law of thermodynamics? Starting with the definition
ΔStotal=ΔSsurr+ΔSsys
we would first like to get rid of ΔSsurr. How can a chemical reaction (a change in the system) affect the entropy of the surroundings? Because most reactions are either exothermic or endothermic, they are accompanied by a flow of heat qp across the system boundary. The enthalpy change of the reaction ΔH is defined as the flow of heat into the system from the surroundings when the reaction is carried out at constant pressure, so the heat withdrawn from the surroundings will be –qp which will cause the entropy of the surroundings to change by –qp/T=–ΔH/T . We can, therefore, rewrite Equation as
ΔStotal=−ΔHT+ΔSsys
Multiplying each side by −T, we obtain
−TΔStotal=ΔH−TΔSsys
which expresses the entropy change of the world in terms of thermodynamic properties of the system exclusively. If −TΔStotal is denoted by ΔG, then we have Equation which defines the Gibbs energy change for the process.
Since most chemical and phase changes of interest to chemists take place under such conditions, the Gibbs energy is the most useful of all the thermodynamic properties of a substance, and it is closely linked to the equilibrium constant.