Review of differential equations:

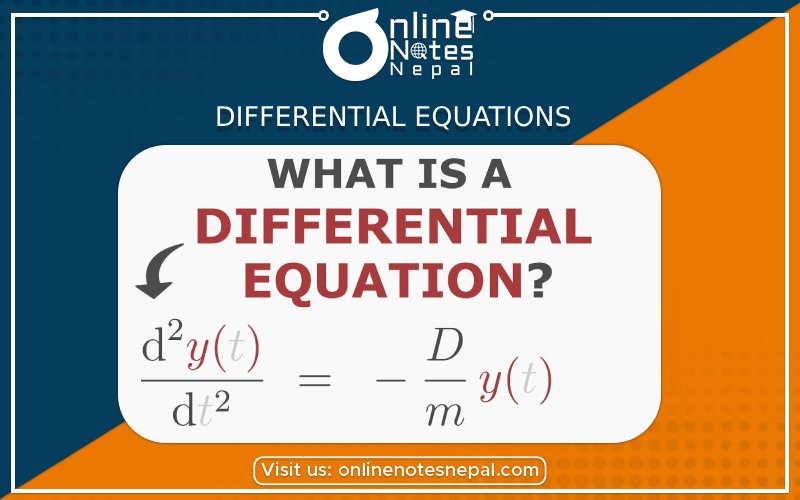
A review of differential equations involves revisiting the fundamental concepts, types, methods of solution, and applications of differential equations. Differential equations are equations that involve one or more derivatives of an unknown function. They are widely used in various fields of science, engineering, and mathematics to model and analyze dynamic systems and phenomena.

Here are some key points to include in a review of differential equations:

Basic Concepts:

- Definition of a differential equation.

- Order and degree of a differential equation.

- Types of differential equations: Ordinary Differential Equations (ODEs) and Partial Differential Equations (PDEs).

- Initial value problems (IVPs) and boundary value problems (BVPs).

1. Types of Differential Equations:

- First-order ODEs: Separable, Linear, Exact, and Homogeneous equations.

- Second-order ODEs: Linear with constant coefficients, Homogeneous, and Inhomogeneous equations.

- Systems of ODEs: Formulation and methods of solution.

2. Methods of Solution:

- Analytical methods: Integrating factors, Substitution, Variation of parameters, Laplace transform, etc.

- Numerical methods: Euler's method, Runge-Kutta methods, Finite difference methods, etc.

3. Existence and Uniqueness of Solutions:

- The existence and uniqueness theorem for first-order ODEs.

- Conditions for the existence and uniqueness of solutions for higher-order ODEs.

4. Laplace Transform:

- Definition and properties of the Laplace transform.

- Solving ODEs using the Laplace transform.

- Inverse Laplace transform and its application.

5. Partial Differential Equations (PDEs):

- Classification of PDEs: Elliptic, Parabolic, and Hyperbolic.

- Examples of PDEs and their physical interpretations.

- Boundary conditions and initial conditions for PDEs.

6. Applications:

- Differential equations in physics, engineering, biology, economics, and other fields.

- Modeling population dynamics, heat conduction, electrical circuits, mechanical systems, and more.

7. Stability and Phase Portraits:

- Stability analysis of ODEs and equilibrium points.

- Phase plane analysis and phase portraits.

8. Numerical Methods:

- Introduction to numerical techniques for solving differential equations.

- Euler's method, Runge-Kutta methods, Finite difference methods, etc.

9. Advanced Topics (Optional):

- Series solutions of ODEs.

- Fourier series and Fourier transforms for solving PDEs.

- Green's functions and eigenvalue problems.

A comprehensive review of differential equations will provide a solid foundation for understanding and tackling various problems in applied mathematics, engineering, and science. It is essential to practice solving a variety of differential equations using different methods to gain proficiency in the subject.